What if a conductor moves in a magnetic field? As a conductor moves, the charges within the conductor are in motion and we all know that magnetic field exerts force on moving charges.
We have a conductor of length \(L\) which moves in a uniform magnetic field \(\vec B\) as shown in Figure 1 below. As the conductor is moved to the right with constant velocity \(\vec v\), the charges within the conductor are also in motion and the magnetic field exerts forces on moving charges.
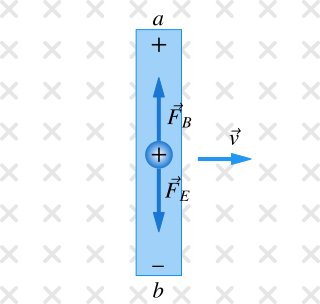
The charges in the conductor are separated which creates an electric field \(\vec E\) and this electric field exerts opposite force on the moving charges. After some time, the magnetic and electric forces balance each other and no further separation of charge takes place.
If \(q\) is the charge and when the electric and magnetic forces cancel each other, you know \(qE = qvB\) where \(v\) is the speed of the conductor. The potential difference between the ends of the conductor, that is potential of \(a\) with respect to \(b\), is
\[V_{ab} = \mathcal{E} = EL = vBL \tag{1} \label{1}\]
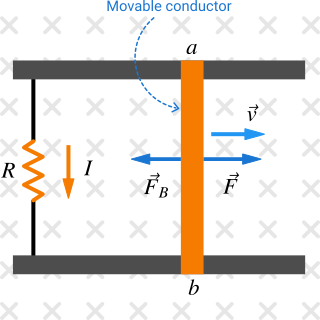
If the conductor is connected to form a complete circuit, the potential difference is \(V_{ab}\) is the emf in the circuit. For example, the ends of the conductor can slide on the two straight conductors as shown in Figure 2. The movable conductor can slide back and forth and hence can induce emf in the circuit.
The above Equation \eqref{1} is valid with Faraday's law. For example, let the conductor moves a distance \(s\) to the right. Now the magnetic flux is \(\Phi_B = BLx\). The Faraday's law gives
\[\mathcal{E} = -\frac{d\Phi_B}{dt} = - BL \frac{dx}{dt} = - BLv \tag{2} \label{2} \]
The negative sign in Faraday's law is the Lenz's law, that is the induced emf has direction such as to oppose the cause of the emf. Do not confuse with Equations \eqref{1} and \eqref{2} with or without the negative sign.
If \(\vec F\) is force applied to move the conductor to the right as shown in Figure 2, let's determine the power input. Since the conductor is moving with constant velocity \(\vec v\) and you know from Newton's first law that a constant velocity is the equilibrium condition. And we know that there is opposite magnetic force on the conductor to the left according to Lenz's law.
\[ P = F v\]
As the velocity to the right is constant, the external force \(\vec F\) and magnetic force \(\vec F_B\) must be equal to each other and therefore \(F_B = F = ILB\) and the power input is
\[P = ILBv\]
You know that \(I = \mathcal{E}/R\) and \(\mathcal{E} = vBL\) from Equation \eqref{1}, the power is
\[P = \frac{\mathcal{E}^2}{R} \tag{3}\]
The work done by the external force is converted to electrical energy. The power input to the circuit by the external force is transferred to the resistor.
Motional Emf and Lenz's Law
As we know in Lenz's law that it gives the direction of induced emf. Lenz's law enforces the law of conservation of energy. In Figure 2, the movable conductor is moving to the right with constant velocity. If the induced emf has clockwise direction instead of anticlockwise direction, the conductor will accelerate further and will cause more current and will accelerate even more and more current and so on. This violates the law of conservation of energy.
As the movable conductor slides back and forth, the direction of induced emf changes continuously. The movable conductor acts like a battery with emf equal to the induced emf and its polarity changes continuously. Therefore the direction of current changes accordingly. The current whose direction changes continuously in an electric circuit is called alternating current. The apparatus in which the alternating current is generated by sliding the conductor back and forth is called slidewire generator.





