Similar to mechanical power in Mechanics, electric power is the rate of energy transfer in an electric circuit. Here we talk about the energy and power relationships in an electric circuit. If there is a fixed potential difference in a circuit element that acts as the energy source, that is the source of emf, the charges flow in the circuit from higher to lower potential energy.
The potential energy decreases as the charge flows in a circuit. The decreased potential energy is transferred to the resistance of circuit and converted to thermal energy. The decreased potential energy is used in vibrating the atoms more and more and hence converted to thermal energy. The thermal energy is then transferred to the surroundings.
Energy is never dissipated. There is a high chance of hearing "energy is dissipated" from various sources which means energy is disappeared but energy does not disappear. Energy is converted or transferred from one form to another. In the sense of "energy is wasted" while saying energy is dissipated, it may make some sense but we can be better on this by saying energy is delivered or transferred and converted to some other form of energy. Getting used to good terminology helps in Physics for the long run!
An external resistance connected to an electric circuit is also called load resistance. The load resistance can be provided by anything such as a light bulb or any other electric appliance. In an electric circuit we are more interested to know the rate of energy transferred, that is the electric power into the load resistance.
ELECTRIC POWER: The rate of electrical energy transfer to a load is called electric power.
Here the load can mean anything that gets energy; you'll know what it means later. We often neglect resistance of wires but real wires always have resistance. If there is a resistor in a circuit, the resistance of the wires is however negligible in comparison to the external resistance.
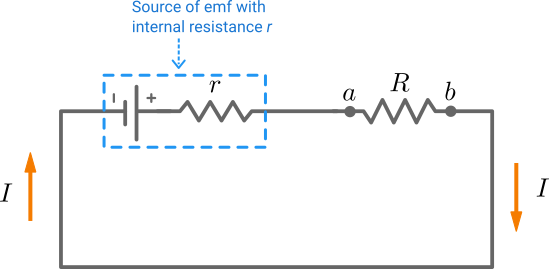
We consider an electric circuit with load resistance \(R\) as shown in Ohm's law, that is the relationship V= IR (this relationship may not always be Ohm's law), and the potential difference across the resistance, that is potential of point \(a\) with respect to the potential of point \(b\) is \(V_{ab} = IR\). If the charge \(dq\) flows through the resistance \(R\) in time \(dt\), the current is \(I = dq/dt\).
Note that current is the same throughout the circuit. You may suspect the current is only for the resistance \(R\) as we just calculated current in a resistance and it seems to a common sense that the current is for the resistance only but that is not correct. The same current exists in an entire circuit no matter where it is calculated, that is in a resistor or in a source or in a wire element.
Several misconceptions about current exist, such as "current comes out of a battery" and "used up" in the resistance. The "current comes out of a battery" is misleading as a battery does not provide current but establishes an electric field in the circuit. And current is never used up in a circuit. It is the same in the entire circuit and depends on the total resistance the circuit has. Similar misconception is current comes out of one terminal of a battery and ends at another terminal.
The change in potential energy across the resistance, that is the electrical work done across the resistance is \(V_{ab}dq\). We know that the electric power is the rate of electrical work done, that is
\[P = V_{ab}dq/dt = V_{ab}I \tag{1} \label{1}\]
The power is being delivered to a resistance means the resistance is getting potential energy at a particular rate. The energy delivered to the resistance is converted to heat energy which is then transferred by different mechanisms of heat transfer. The heat energy increases in the same rate as rate of potential energy transferred to the resistance.
Electric Power Input to a Load
As you already know, a load in an electric circuit can be anything that uses electrical energy, that is it can be a bulb or resistor or any other electrical appliance. We now just consider a resistor of resistance \(R\) as our load in our electric circuit.
We now take our equation \eqref{1} and we know the relationship \(V_{ab} = IR\) sometimes called Ohm's law (calling this relationship Ohm's law is really a bad practice). Then we can write,
\[P = I^2 R \tag{2} = \frac{{V_{ab}}^2}{R}\]
The above equation tells us that the electric power, that is the rate of energy transfer to a resistance is inversely proportional to the resistance for a fixed voltage (potential difference). In other words the electric power input to a load is inversely proportional to the resistance of the load for a fixed voltage. This makes sense because greater the resistance, less the current and less charge flows through the resistance per unit time for a fixed voltage and less electric power.
Electric Power Output of a Source of Emf
Considering our circuit has only a load resistance \(R\) and internal resistance of our source of emf \(r\), that is neglecting the resistance of wires, we know the terminal voltage \(V_{ab}\) is \(V_{ab} = \mathcal{E} - Ir\). So, the power from Equation \eqref{1} can be rewritten as,
\[P = \mathcal{E}I - I^2r \tag{3}\]
So, it is clear that the power output of a source of emf is lesser by the term \(I^2r\). It means some of the energy is transferred to the internal resistance of the source of emf such as warming up a battery.
Electric Power Input to a Source of Emf
Here we give electric power input to a source of emf, such as a rechargeable battery so that the energy we supplied can be later recovered as electrical energy. When we give electric power input to rechargeable batteries, the electrical energy we supply is converted to chemical energy again (that chemical energy was decreased before into electrical energy) and we say the battery is charged or charging. That chemical energy can again be recovered as electrical energy.

In Figure 2 above source \(A\) is delivering electric power to source \(B\) of emf \(\mathcal{E}\) and internal resistance \(r\). The emf of source \(A\) is greater than the emf of source \(B\). But here the terminal voltage of source \(A\) plays the role of delivering electric power. We consider the internal resistance of source \(A\) is small enough, so the terminal voltage \(V_{ab}\) of source \(A\) is greater than the emf of source \(B\).
When the electric power is being delivered to the source \(B\), the direction of electric current is opposite to the direction of current when the source \(B\) delivers power to a load (resistance) if connected to it. In this case, if \(I\) is the current, \(V_{ab}\) is
\[V_{ab} = \mathcal{E} + Ir\]
Here the work is being done on source \(B\). From Equation \eqref{1} we know the electric power input to the source \(B\) is
\[P = \mathcal{E}I + I^2r \tag{4} \label{4}\]
Notice the plus '\(+\)' sign instead of the '\(-\)' sign in above equation. When you deliver power to a source of emf \(\mathcal{E}\) and internal resistance \(r\) to store electrical energy in some other form of energy such as chemical energy, the total power input to the source is \(\mathcal{E}I + I^2r\). For example, for rechargeable batteries, the additional power given by the term \(I^2r\) is converted to thermal energy which is used in warming up the battery and that power is wasted in practical sense.





