How electrical conduction works in metals? We still need quantum Physics to agree with the experimental results but the classical approach can also be beneficial to some extent.
Here we consider a model for electrical conduction in metals also called classical model for the electrical conduction in metals which ultimately focuses on the validity of Ohm's law. Although this model has limitations, we can still use this model to describe the electrical conduction in metals obeying Ohm's law. We are talking about the most basic theory of metallic conduction by classical model.
The analysis of this model includes some basic assumptions. A metal consists of particle-like free electrons also called conduction electrons and their corresponding massive stationary positive ions. The atoms of the metal contribute one of more free electrons which can freely move in the metal.
If an electric field is applied to a metal, the conduction electrons experience a force and hence the electric field does work on the electrons. This is similar to a ball falling along a ramp with obstacles under the action of gravity as shown in Figure 1 below.
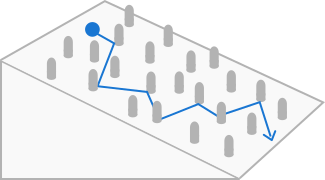
In electrostatic situation, that is in the absence of electric field the electrons move randomly in any direction. The random motion of electrons does not lead them anywhere, that is the motion is random and on average the net motion towards a particular direction is zero. The random motion of electrons is similar to the random motion of ideal gas molecules in a container.
What we determine from this model is the resistivity and we determine the drift velocity first.
In the presence of electric field, the electric field does work on electrons. The electric field applies force \(q\vec E\) on each electron where \(q=-e\). The force causes acceleration but due to the collisions with the massive stationary ions, the net effect is the slow drift velocity opposite to the direction of electric field. The speed of random motion of electron is very high which is of the order of \(10^6 \text{m/s}\) but the slow drift speed is of the order of \(10^{-4} \text{m/s}\).

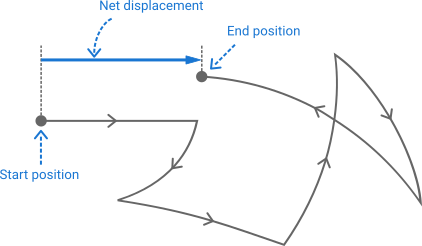
In Figure 2 and Figure 3 the path between collisions is straight line without electric field but if the electric field is present, the path between collisions is somewhat curved due to the force caused by the electric field. When the electric field is present there is also a net displacement.
In the presence of electric field, there are both the random motion and the slow drift of electrons. The work done by the electric field on electrons is transferred to the massive stationary positive ions of the metal, that is the kinetic energy gained by electrons is transferred to the ions and hence spent in increasing the internal energy of the metal.
The electric field is absent and the average velocity of electrons is zero. When an electric field \(\vec E\) is applied, an electron of mass \(m_e\) experiences force \(q\vec E\) or \(-e\vec E\). Let the initial velocity of an electron is \(\vec v_i\). If \(\tau\) (Tau) is the average time interval between collisions, the final velocity of the electron after that time is
\[\vec v_f = \vec v_i + \vec a \tau\]
Now we determine the average velocity of electrons due to the electric field. You know that the electrons were initially in random motion in any direction and the average of initial velocity of all electrons is zero, that is average of \(\vec v_i\) is zero. So, we have from the above equation,
\[\begin{align*} \vec v_\text{av} &= \vec a \tau \\ \text{or,} \quad \vec v_\text{av} &= \frac{q\vec E \tau}{m_e} \tag{1} \label{1} \end{align*}\]
The average time \(\tau\) depends on the size of positive ions and the number of electrons per unit volume. The average velocity \(\vec v_\text{av}\) remains the same even after time \(t = \tau\). After the time \(\tau\), the force tries to increase the velocity of electrons but the collisions with the massive stationary ions decrease the velocity and the average velocity does not change. The average velocity \(\vec v_\text{av}\) is also called drift velocity \(\vec v_{d}\), so
\[\vec v_d = \frac{q\vec E \tau}{m_e} \tag{2} \label{2}\]
The equation of current density is \(\vec J = nq\vec v_d\) and \(n\) is the number of electrons per unit volume. So, we know the expression for the drift velocity from above Equation \eqref{2} and the current density is
\[\vec J = \frac{nq^2\vec E}{m_e} \tau \tag{3} \label{3}\]
We know from Ohm's law, \(\vec J = \sigma \vec E\) where \(\sigma \) is the conductivity. The reciprocal of conductivity is resistivity, that is resistivity \(\rho\) is \(\rho\) = \(1/\sigma\). So, comparing Equation \eqref{3} with the expression from Ohm's law, we have the resistivity as
\[\rho = \frac{m_e}{nq^2\tau} \tag{4} \label{4}\]
You know from the beginning of this model that this model does not include anything about the temperature dependence of resistivity. From the above Equation \eqref{4} if \(n\) and \(\tau\) do not change, the resistivity is independent of the electric field. The expression of the resistivity using this model is based on the validity of the Ohm's law.
As the temperature of a metal increases, the massive positive ions vibrate more and more about their equilibrium position and increase more collisions and hence the resistivity increases, that is greater the collisions, greater the resistivity.
To address the facts regarding the temperature dependence of resistivity we need to develop the classical model into quantum-mechanical model for electrical conduction, so the values of resistivities agree with the experimental values. It means we need to consider the wave-like behavior of particles.
Considering the wave-like nature of electrons, if a crystal is perfect, that is no atom is misplaced, the electrons get the free way to move without obstacles and the current becomes infinite (it is possible because electrons are wave-like). Even in a perfect crystal, if you increase the temperature, the atoms start to vibrate about their equilibrium position which increases the chance of collision and electrons collide with the atoms thereby increasing the resistivity. This information is not included in the classical model for electrical conduction in metals which is stuck with the empirical Ohm's law.





