Lenz's law is entirely related to Faraday's law. It is far more easier to use Lenz's law to determine the direction of induced emf.
Lenz's law tells us about the direction of induced emf and that's all. It can be stated as
LENZ'S LAW: The induced emf has a direction to oppose the change in magnetic flux.
Induced emf means induced current and the induced current has its own magnetic field. Now the catch is that the magnetic field must be in a direction that opposes the change in magnetic flux. In Figure 1, the magnet is moving towards the loop.

Now for the case in Figure 1 the lenz's law tells us that the direction of magnetic field must be opposite to the magnetic field of the bar magnet so that the change in magnetic flux can be opposed. In other words, the near side of the coil acts as the north pole; like poles repel and it is harder to move the magnet towards the coil, that is the change in magnetic flux is opposed. And now we know the direction of magnetic field on the axis of the loop, that is opposite to that of the bar magnet.
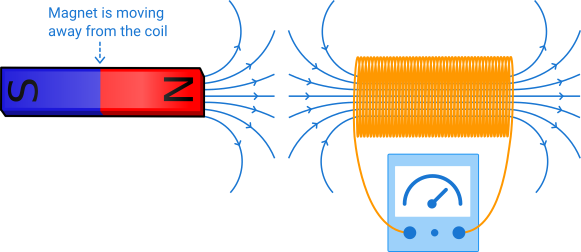
In case of Figure 2, the magnet is moving away from the coil. In this case the induced magnetic field in the coil tries to pull the magnet towards the coil, that is, it opposes the motion away from the coil and hence opposes the change in flux. And to do that the magnetic field in the coil must be in the same direction as that of bar magnet (the side of coil near the magnet acts as the south pole and you know unlike poles attract).
Once you know the direction of magnetic field according to Lenz's law, you can easily find the direction of induced current and hence emf, that is you can use the right hand rule (use thumb to point towards the direction of magnetic field and the curled fingers automatically give the direction of induced emf and current in the loop).
Another example of Lenz's law is illustrated by Figure 3. In this case the movable conductor can slide on the conducting rods and the conducting rods complete the circuit. As the movable conductor slides on the rods, emf is induced in the circuit. For example, the movable conductor slides towards the right. In which direction the induced emf should be?

The emf has a direction so that the induced magnetic field can oppose the motion of the moving conductor. And in this case, the induced magnetic field must be out of the screen so that it can apply force towards the left opposing the motion of the moving conductor towards the right, and hence the direction of induced emf or current is anticlockwise.
Lenz's Law and the Law of Conservation of Energy
Lenz's law is in accordance with the law of conservation of energy. In other words Lenz's law expresses the law of conservation of energy. Let's examine the situation in Figure 3 above. If the induced current is in clockwise direction instead of anticlockwise direction, the induced magnetic field exerts force to the right. That further accelerates the conductor and more emf is induced which means more current and more force and it never ends. The work is being done without adding energy. This violates the law of conservation of energy.
Lenz's Law and Circuit Resistance
We have the relationship derived from Ohm's law, that is \(\mathcal {E} = IR\) where \(I\) is the induced current and \(R\) is the circuit resistance. The greater the circuit resistance, the less the induced current. And if the induced current is less, the induced magnetic field to oppose the change in flux is less and it's easier to change flux. The less the circuit resistance, the greater the induced current and greater the induced magnetic field and it's difficult to change the flux.
In case of insulators, almost no current is induced because of the very high resistance. In case of superconductors, the resistance is zero and once the current is induced, the current persists even if there is no induced emf, that is no change in magnetic flux.





