The surface on which the potential is the same at every point on the surface is equipotential surface. Since the potential is the same at every point on the surface, the change in potential on an equipotential surface is zero.
If an element charge moves through a small displacement \(d\vec s\) on an equipotential surface within electric field \(\vec E\), the change in potential is \(dV = -\vec E \cdot d\vec s = 0\). Therefore, the electric field must be perpendicular to an equipotential surface.
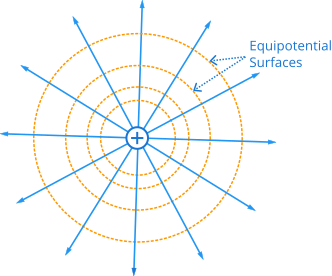
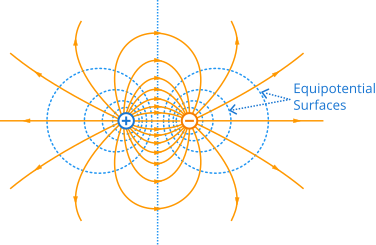
The equipotential surfaces are drawn in Figure 1 and Figure 2 in a way that the potential differences between the adjacent pairs are the same. Note that the equipotential surfaces are three dimensional.
The equipotential surfaces in Figure 2 are closer on the right side of positive charge or on the left side of negative charge where the electric field is relatively stronger than on the right side of negative charge or on the left side of positive charge, that is the electric field is stronger between the charges and greater work is done over a relatively small displacement.
On the right side of negative charge or left side of positive charge, the electric field is relatively weaker and therefore the equipotential surfaces are farther apart. This concludes that the electric field may not necessarily be the same over an equipotential surface.





