You may know that there is a difference between a moving charge and a stationary charge. The difference is that a moving charge has both electric and magnetic fields but a stationary charge has only electric field. Electromagnetism is all about the study of these forces (electric and magnetic forces). The result of motion of a charge moving in a magnetic field very interesting.
In Figure 1 the magnetic field is directed inward into the screen (you are reading in the screen of a computer or a smart phone) represented by the cross (X) signs. Note that the magnetic field directed into the screen is represented by a collection of cross signs and those directed out of the screen towards you are represented dots (see Figure 2).

Think this way, an arrow is moving towards you and what you notice is the tip of the arrow (represented by dot), that is the same as moving outward from the screen (towards you). If you look at the arrow moving away from you, you notice the tail of the arrow (represented by cross), that is moving into the screen (moving away from you).
In Figure 3 a charge \(q\) is moving in the magnetic field \(\vec B\) with speed \(v\). I considered the charge is moving with speed \(v\) not with velocity \(\vec v\) because the velocity changes continuously, that is the charge's direction is changing continuously. But if you consider a particular instant of motion, it has a velocity vector \(\vec v\). As the charge moves the magnetic field exerts magnetic force on the charge and its direction is perpendicular to the plane containing \(\vec v\) and \(\vec B\).
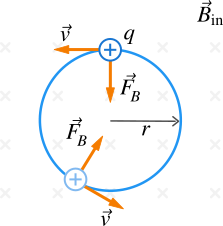
Since the magnetic force is directed perpendicular to the plain containing \(\vec v\) and \(\vec B\), that is the magnetic force \(\vec F\) is always perpendicular to \(\vec v\), the charge moves in a circle of arbitrary radius \(r\) (see fig). It is because the direction of force is always perpendicular meaning the force is always directed to the center of the circle. So, the magnetic force also provides the centripetal force to the charge. Let's see what happens next.
The magnitude of magnetic force on the charge is
\[F =|q|vB\sin \theta = qvB \tag{1} \label{1}\]
where \(\theta\) is the angle between \(\vec v\) and \(\vec B\) but the angle is always a right angle, so \(\sin \theta = 1\). The absolute value of charge |q| is used because we are only considering the magnitude of magnetic force. And already noted, this force provides the centripetal force to the charge. If the charge has mass \(m\), the expression of the centripetal force on the charge is
\[F = \frac{mv^2}{r} \tag{2} \label{2}\]
Equating Equations \eqref{1} and \eqref{2}, and solving for \(r\), you get
\[r = \frac{mv}{|q|B} \tag{3} \label{3}\]
So, what we got here is an expression for the radius of the circle in which the charge moves under the action of magnetic force. You can easily understand the proportionality of the radius to other related quantities from the above equation. If the charge is negative the rotation is clockwise.
The angular speed \(\omega\) is related to the linear speed \(v\) and radius \(r\), that is \(\omega = v/r\), so the angular speed using Equation \eqref{3} is
\[\omega = \frac{|q|B}{m} \tag{4} \label{4}\]
You know that the frequency \(f\) of the rotation is \(\omega / 2\pi\). And you got
\[f = \frac{|q|B}{2\pi \, m} \tag{5} \label{5}\]
The above Equation \eqref{5} suggests that the frequency of rotation does not depend on the radius of the circle and speed (linear) of the charge and it is also called cyclotron frequency. Cyclotron is a device where elementary particles are accelerated such as protons at high speeds.
The angular speed is also cyclotron frequency! Note the cyclotron is just a device. So it is not strict to call only the frequency of rotation as cyclotron frequency. Some physicists also call angular speed (angular frequency) the cyclotron frequency.
So, we can change the linear speed and radii without affecting the angular speed or frequency. The Equation \eqref{5} also suggests we can change the cyclotron frequency by simply changing the magnetic field.
The angle between magnetic field and velocity vector at each instant of motion of the charged particle is the right angle. But if the angle is not a right angle there is also a component of velocity vector parallel to the magnetic field. There is no magnetic force for the motion parallel to the magnetic field, this parallel component remains constant and the motion of charged particle is helical, that is the charge moves in a helix as shown in figure below.
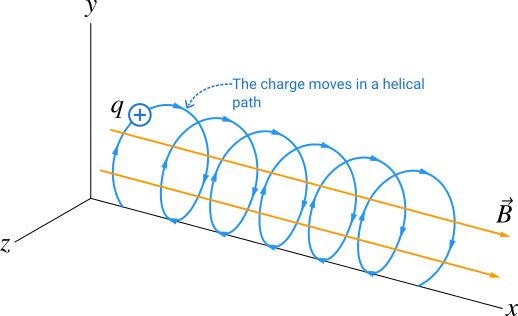
Storing charged particles (ionized gas) in a magnetic field has a huge importance. For example you can hold ionized gas of very high temperature such as \(10^6 \text{K}\) in a magnetic bottle which can destroy any material if comes in contact with such a high temperature.





