What if the velocity changes with time? Acceleration is the rate of change of velocity also called linear acceleration similar to the rate of change of displacement is velocity. Here we illustrate the linear acceleration different from angular acceleration of angular motion. A general trend is that if we simply call acceleration we mean linear acceleration. And if we mean angular acceleration, we use the word "angular" to distinguish it from the linear motion.
We illustrate the acceleration with an example here. In Figure 1 a car has velocity \({{v}_{1}}\) at point \({{p}_{1}}\) and \({{v}_{2}}\) at point \({{p}_{2}}\) along x-axis. The earlier velocity \({{v}_{1}}\) is the initial velocity \({{v}_{i}}\) and the final one is \({{v}_{2}}\) which is \({{v}_{f}}\). The subscripts \(i\) and \(f\) represent the initial and final velocities respectively.
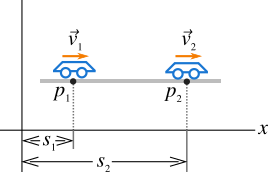
The change in velocity is the final velocity minus the initial velocity, that is, \(\Delta v={{v}_{2}}-{{v}_{1}}={{v}_{f}}-{{v}_{i}}\). The time of the car at point \(p_1\) is \(t_1\) and at point \(p_2\) is \(t_2\). The time taken by the car to move from point \(p_1\) to the point \(p_2\) is \(\Delta t={{t}_{2}}-{{t}_{1}}\). We express the rate of change in velocity as,
\[{{a}_\text{av}}=\frac{{{v}_{f}}-{{v}_{i}}}{\Delta t}=\frac{\Delta v}{\Delta t} \tag{4} \label{4}\]
Notice that the subscript "av" represents the average acceleration. The acceleration in Equation \eqref{4} is average acceleration because we don't know the rate of change of velocity is constant or not throughout the motion.
The whole process going here is, suppose the velocity at point \({{p}_{1}}\) is \(5\text{m/s}\) and after \(1\text{s}\) it is \(10\text{m/s}\), and again after 1s it is \(12\text{m/s}\). The acceleration during the first \(1\text{s}\) is \(\frac{(10-5)\text{m/s}}{1\text{s}}=5\text{m/}{{\text{s}}^{\text{2}}}\) and during the next \(1\text{s}\) is \(\frac{(12-10)\text{m/s}}{1\text{s}}=2\text{m/}{{\text{s}}^{\text{2}}}\), so the average of these two accelerations is \(\frac{(5+2)\text{m/s}}{2}=3.5\text{m/}{{\text{s}}^{\text{2}}}\) which is the same as \(\frac{(12-5)\text{m/s}}{2\text{s}}=3.5\text{m/}{{\text{s}}^{\text{2}}}\).
When \(\Delta t\) approaches zero, the acceleration is instantaneous acceleration similar to instantaneous velocity. The instantaneous acceleration is the acceleration at a particular instant of time.
The instantaneous acceleration gives the acceleration at any point at any instant of time, so we mean the term instantaneous acceleration as a single word "acceleration". For example, if we say that a car has acceleration \(3\text{m/}{{\text{s}}^{\text{2}}}\) at any point, that acceleration is instantaneous acceleration at that point.
The instantaneous acceleration is the average acceleration in Equation \eqref{4} in the limit that \(\Delta t\) approaches zero (the point \({{p}_{1}}\) is very close to the point \({{p}_{2}}\)).
\[{{a}_{\text{ins}}}=a=\underset{\Delta t\to 0}{\mathop{\lim }}\,\frac{{{v}_{f}}-{{v}_{i}}}{\Delta t}=\underset{\Delta t\to 0}{\mathop{\lim }}\,\frac{\Delta v}{\Delta t}=\frac{dv}{dt} \tag{5} \label{5}\]
The subscript \(\text{ins}\) represents instantaneous for instantaneous acceleration which is not always necessary.


The graph of \({{v}_{x}}-t\) is always a straight line if the acceleration is constant indicating the constant slope. You may know that the slope at any point on the \({{v}_{x}}-t\) graph gives the instantaneous acceleration at that point. In Figure 6 the slope is constant and acceleration is the same (constant) throughout the motion. What if the acceleration is not constant? You will find the result of \({{v}_{x}}-t\) graph to be some kind of curve and the slope at any point on the curve gives the acceleration at that point as in Figure 7
The acceleration is dangerous to humans not the velocity. The Newton's first law of motion tells us that a constant velocity is the equilibrium condition and it is safe. The only thing you need to worry about is the changing velocity. Learn more on "why higher acceleration is dangerous".
The rate of change of displacement is velocity. The rate of change of velocity is the acceleration. What if the acceleration changes with time. We call the rate of change of acceleration as jerk or also called jolt. The rate of change of jerk is called jounce or snap. You may have felt jerk at the time of learning to drive a car. Inexperienced drivers provide jerk to the car by inappropriately applying the clutch and accelerator.





